- UID
- 97649
- 帖子
- 1177
- 主题
- 755
- 注册时间
- 2008-5-20
- 最后登录
- 2011-12-22
|
8#
 发表于 2009-6-30 17:56
发表于 2009-6-30 17:56
| 只看该作者
106. A U.S. investor has purchased a tax-exempt 5-year municipal bond at a yield of 3.86 percent which is 100 basis points less than the yield on a 5-year option-free U.S. Treasury. If the investor’s marginal tax rate is 32 percent, then the yield ratio are closest to:
A. 0.79
B. 1.26
C. 5.68
Answer: A
“Understanding Yield Spread,” Frank J. Fabozzi
2009 Modular Level I, Volume 5, pp. 335-336
Study Session 16-63-e, i
Compute, compare, and contrast the various yield spread measures.
Compute the after-tax yield of a taxable security and the tax-equivalent yield of a tax-exempt security.
Yield ratio = (yield on tax-exempt bond) / (yield of US Treasury) = 3.86 / (3.86 + 100bp) = 3.86 / 4.86 = 0.79
107. An analyst has gathered the following information provided in the table below:
 eriod eriod |
Years |
U.S. Treasury Spot Rate
(%) |
Credit Spread
(%) |
1
2
3
4
5 |
1
2
3
4
5 |
3.00
3.50
4.00
4.50
5.00 |
0.20
0.30
0.40
0.50
0.60 | Based on the information provided in the table, the current market price of a $1,000 par value, option-free, 0 percent coupon corporate bond maturing in 5 years is closest to:
A. $758.70.
B. $781.20.
C. $804.44.
Answer: A
“Introduction to the Valuation of Debt Securities,” Frank J. Fabozzi
2009 Modular Level I, Volume 5, pp. 366-371
Study Session 16-64-e
Compute the value of a zero-coupon bond.
The appropriate discount rate is 5.6% = 5% + 0.6%. The semiannual discount rate is 2.8%. The price of the bond using semiannual discounting is:
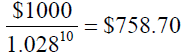
108. An analyst gathered the following information about a portfolio comprised of
three bonds:
| Bond |
 rice ($) rice ($) |
 ar Amount ar Amount
Owned |
Duration |
| A |
102.000 |
$7 million |
1.89 |
| B |
94.356 |
$5 million |
7.70 |
| C |
88.688 |
$3 million |
11.55 | Assuming there is no accrued interest, then the portfolio duration is closest to:
A. 5.55 years.
B. 5.76 years.
C. 6.82 years.
Answer: A
“Introduction to the Measurement of the Interest Rate Risk,” Frank J. Fabozzi
2009 Modular Level I, Volume 5, pp. 468
Study Session 16-66-f
Compute the duration of a portfolio, given the duration of the bonds comprising the portfolio, and explain the limitations of portfolio duration.
Portfolio value = (1.02 × 7 mil) + (0.94356 × 5 mil) + (0.88688 × 3 mil) =14,518,440
Weight, Bond A = 7,140,000 / 14,518,440 = 0.492
Weight, Bond B = 4,717,800 / 14,518,440 = 0.325
Weight, Bond C = 2,660,640 / 14,518,440 = 0.183
Portfolio duration = (0.492 × 1.89) + (0.325 × 7.70) + (0.183 × 11.55) = 5.55 |
|

