AIM 1: Define individual VAR, marginal VAR, incremental VAR and diversified portfolio VAR.

1、After regressing the return of a position in the portfolio on the return of the entire portfolio, the slope coefficient (beta) can be used in the marginal VAR formula. Which of the following is the best representation of that formula? Marginal VAR equals:

A)  [attach]13943[/attach]

B)  [attach]13944[/attach]

C)  [attach]13945[/attach]

D)  [attach]13946[/attach]

[此贴子已经被作者于2009-7-2 10:16:09编辑过]

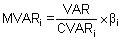
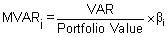
The correct answer is D

This is the correct formula.

2、Marginal VaR is best described as the change in VaR that results from:

A) subtracting idiosyncratic VaR from total VaR.

B) changing the weight on an existing position by one unit.

C) removing an existing asset from a fund. 

D) adding a new asset to a fund.

The correct answer is B

Marginal VaR or MVaRi, is the change in the portfolio VaR per unit change in the weight in Fund i. The following is an expression that represents the marginal VaR of a portfolio:

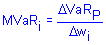
3、With respect to marginal VaR (MVaR), which of the following is FALSE?

A) MVaR is the amount of risk a fund contributes to a portfolio. 

B) MVaR is an approximation based upon a small change in a fund’s portfolio weight.

C) MVaR can be positive or negative.

D) MVaR is a rate of change measure.

The correct answer is A

MVaR is defined as the change in the portfolio VaR per unit change in the weight in a fund. The amount of risk a fund contributes to a portfolio is the definition of component VaR (CVaR).

4、Which of the following is the best interpretation of incremental VaR?

A) It is marginal VaR where the initial weight is zero.

B) It is the VaR for the first step into the tail beyond the VaR level.

C) It is the VaR for liquidating a position in increments. 

D) It is the amount of risk a particular fund contributes to a portfolio of funds.

The correct answer is A

Incremental VaR (IVaRi) is an estimate of the amount of risk a proposed new position in Fund i will add to the total VaR of an existing portfolio.

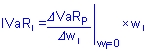
AIM 5: Compute the variance minimizing allocation or best hedge when adding a single risk factor to a portfolio.

When considering increasing the dollar exposure to a given position with a single risk exposure, the best hedge or minimum-variance allocation is represented by:

A) 

 [attach]13949[/attach]

B) 

 [attach]13950[/attach]

C) 

 [attach]13951[/attach]

D) 

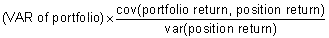
The correct answer is C

In symbols, the representation is either of the following:

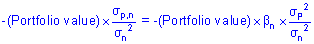
AIM 3: Compute the standard deviation and VAR of an equally weighted portfolio, with equal standard deviations and correlations.

A manager has a portfolio of 25 positions. The returns of the 25 positions have the same standard deviation and the correlations between positions are all equal. The standard deviation of each position is 40% and the correlations are 0.3. If the portfolio’s value is $2 million, using a Z-value of 1.65 the VAR of the portfolio is closest to:

A) $755,981. 

B) $396,000. 

C) $87,636. 

D) $79,200. 

The correct answer is A

Using the formula for the standard deviation of the portfolio:

σ = 22.91%

VAR = 1.65 × 0.2291 × $2,000,000 = $755,981

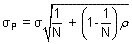
AIM 6: Compute component VAR in a portfolio with a large number of positions and use it to decompose VAR.

1、For a portfolio with a large number of relatively small positions, the component VAR of a given position would probably be closest to:

A) the position’s marginal VAR divided by the value invested in the position. 

B) the position’s marginal VAR multiplied by the value invested in the position. 

C) the position’s marginal VAR multiplied by the beta of the position with the overall portfolio. 

D) the position’s marginal VAR divided by the beta of the position with the overall portfolio. 

The correct answer is B

In a large portfolio with many positions, the approximation is simply the marginal VAR multiplied by the dollar weight in position “i”: CVARi = (MVARi) × (wi × P) where P is the value of the portfolio, wi is the weight in the portfolio.

2、In a portfolio of three currencies, the relationship between the component VAR and the individual VAR for each currency is likely to be what?

A) The individual VAR is likely to be equal to the component VAR.

B) The individual VAR is likely to be less than the component VAR.

C) There is no relationship between the component VAR and the individual VAR.

D) The component VAR is likely to be less than the individual VAR.

The correct answer is D

Unless assets are perfectly correlated, component VAR will be less than individual VAR.

3、The amount of risk a particular fund contributes by its position in the portfolio of funds is called:

A) liquidity VaR. 

B) marginal VaR. 

C) stress VaR.

D) component VaR.

The correct answer is D

This is the definition of component VaR. It will generally be less than the VaR of the fund by itself because of the diversification of some of the fund’s risk at the portfolio level.

AIM 7: Describe ways we can compute component VARs for a distribution of returns that is not normal or elliptical.

Computing component VAR for a position using the position’s beta with respect to the entire portfolio is appropriate for returns that follow:

A) an elliptical distribution but not a normal distribution. 

B) both an elliptical distribution and a normal distribution. 

C) a normal distribution but not an elliptical distribution. 

D) neither an elliptical distribution nor a normal distribution. 

The correct answer is B

It is appropriate for elliptical distributions, and normal distributions are a subset of elliptical distributions.

AIM 2: For a two-asset portfolio, compute the portfolio VAR when the returns have no correlation and perfect correlation, respectively.

1、Simply adding the VARs for each security in a portfolio to compute the portfolio value at risk (VAR) implies the assumption of:

A) perfect and negative correlation.

B) imperfect and positive correlation.

C) imperfect and negative correlation.

D) perfect and positive correlation.

The correct answer is D

Simply adding the VARs of individual securities to compute the portfolio VAR assumes that there is a correlation of “1” between all the securities. A correlation value of “1”, is perfect and positive. This is called the undiversified VAR.

2、An investor has two stocks, Stock R and Stock S in her portfolio. Given the following information on the two stocks, the portfolio's standard deviation is closest to:

σR = 34%

σS = 16%

rR,S = 0.67

WR = 80%

WS = 20%

A) 8.7%.

B) 2.1%.

C) 29.4%.

D) 7.8%.

The correct answer is C

The formula for the standard deviation of a 2-stock portfolio is:

s = [WA2sA2 + WB2sB2 + 2WAWBsAsBrA,B]1/2

s = [(0.82 × 0.342) + (0.22 × 0.162) + (2 × 0.8 × 0.2 × 0.34 × 0.16 × 0.67)]1/2 = [0.073984 + 0.001024 + 0.0116634]1/2 = 0.08667141/2 = 0.2944, or approximately 29.4%.

3、Which of the following is NOT a primary factor affecting the risk of a portfolio?

A) Total risk for a large portfolio of diversified assets. 

B) The degree to which assets within the portfolio move together.

C) A high degree of concentration in one asset within the portfolio.

D) The volatility of individual assets held within the portfolio.

The correct answer is A

In a diversified portfolio with a large number of assets, the most relevant risk is systematic risk since the unsystematic (i.e., firm-specific risk) gets diversified away. In other words, the unsystematic risks of the individual assets offset each other.

AIM 4: Compute incremental VAR, explain why calculating incremental VAR may be difficult, and give a useful approximation.

1、A portfolio consists of assets A and B. The volatilities are 10% and 20%, respectively. There are $10 million and $5 million invested in them, respectively. If we assume they are uncorrelated with each other, the VAR of the portfolio using Z = 1.65 would be closest to:

A) $2.475 million. 

B) $1.750 million. 

C) $3.500 million.

D) $2.333 million. 

The correct answer is D

We can use matrix notation to derive the dollar variance of the portfolio:

 [attach]13956[/attach]

This value is in ($ millions)2. VAR is then the square root of this value times 1.65: VAR = 1.65 × ($1,414,214) = $2,333,452.

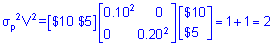
2、A manager is considering adding a new position to a portfolio. The size of the position will be 1% of the portfolio. The manager computes the derivative of the portfolio’s VaR with respect to the change in the weight of the position. Multiplying the value of the derivative times 1% will yield:

A) marginal VaR.

B) incremental VaR.

C) component VaR. 

D) Monte Carlo VaR.

The correct answer is B

Incremental VaR, or IVaRi, is an estimate of the amount of risk a proposed new position in fund i will add to the total VaR of an existing portfolio.

3、The difference between marginal value at risk (MVAR) and incremental value at risk (IVAR) is that:

A) Incremental VAR computes actual changes in VAR for any additions of securities.

B) Incremental VAR only captures changes over small increments.

C) Marginal VAR captures non-linear changes in the portfolio.

D) Marginal VAR only captures changes over large increments.

The correct answer is A

Marginal VAR is an approximation of the changes in the VAR of the portfolio, in response to the addition of one unit (dollar) of a security, and is based on a linear relationship. Like duration, this linear relationship is only accurate for small additions. Incremental VAR computes the actual changes in portfolio VAR for any size additions to the portfolio. Incremental VAR involves the calculation of an entirely new VAR for the portfolio and is used when the changes in VAR cannot be described by a linear function.